

Best explanation of geometric and arithmetic sequences how to#
Equality is only obtained when all numbers in the data set are equal otherwise, the geometric mean is smaller. In this explainer, we will learn how to solve real-world applications of geometric sequences and series, where we will find the common ratio, the t h term explicit formula, the order and value of a specific sequence term, and the sum of a given number of terms. So my goal here is to figure out which of these sequences are arithmetic sequences. They are sequences where each term is a fixed number larger than the term before it. And they are usually pretty easy to spot. In the following exercises, write the first five terms of each sequence with the given first term and common difference. What I want to do in this video is familiarize ourselves with a very common class of sequences. The geometric mean of a non-empty data set of (positive) numbers is always at most their arithmetic mean. 18 Determine if a Sequence is Arithmetic. Main article: Inequality of arithmetic and geometric means Consider an infinite geometric sequence a, ar, ar a / (1 - r), when r < 1 and S diverges when r 1. About Transcript Sal introduces geometric sequences and their main features, the initial term and the common ratio. Geometric proof without words that max ( a, b) > root mean square ( RMS) or quadratic mean ( QM) > arithmetic mean ( AM) > geometric mean ( GM) > harmonic mean ( HM) > min ( a, b) of two distinct positive numbers a and b The tenth term could be found by multiplying the first term by the common ratio nine times or by multiplying by the common ratio raised to the ninth power.N-th root of the product of n numbers Example of the geometric mean: l g. They even have a nifty bit of notation - the exclamation mark. These two sequences converge to the same number, the arithmeticgeometric mean of x and y it is denoted. As you have noticed, it has a recursive definition: a 1, and a na Factorials crop up quite a lot in mathematics. In mathematics, the arithmeticgeometric mean of two positive real numbers x and y is the mutual limit of a sequence of arithmetic means and a sequence of geometric means : Then define the two interdependent sequences (an) and (gn) as. That sequence is the 'factorial' numbers. If you havent already, rewrite the expression as an. If the rule is to multiply or divide by a specific number each. You're right, that sequence is neither arithmetic nor geometric. Does this expression best fit the definition of a Recursive Formula or an Explicit Formula.
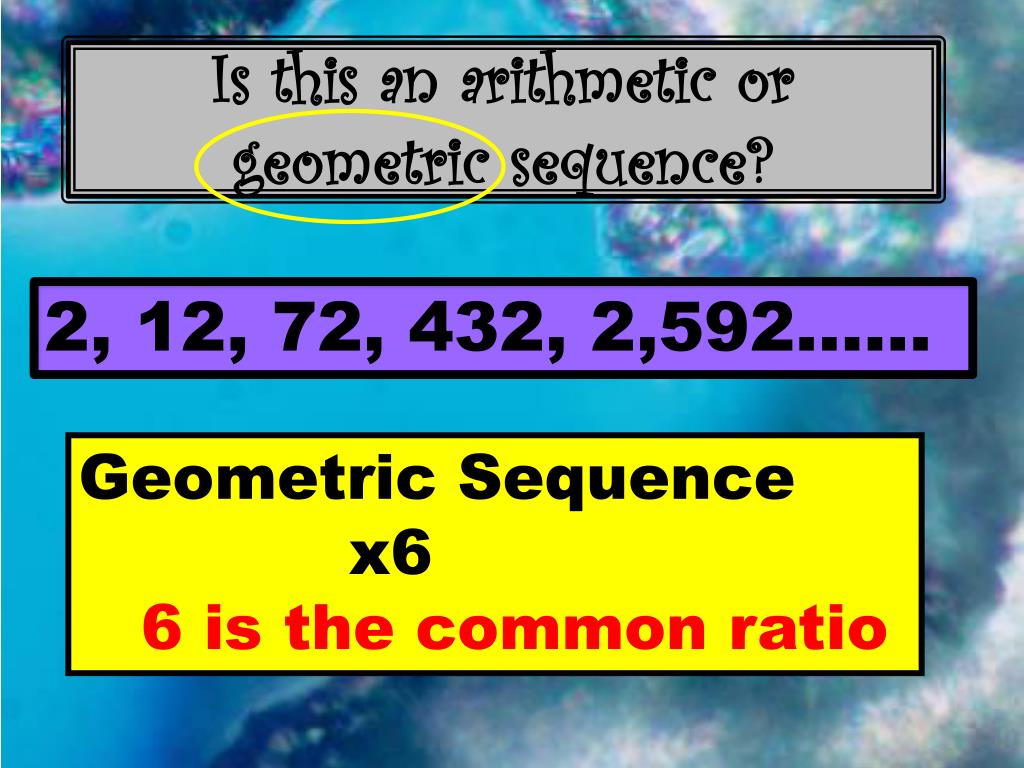
Given the arithmetic sequence 1 x y QGWKHYDOXHVRI x and y. Find the value(s) of y in the geometric sequence: 25 y 1. An arithmetic sequenceA sequence of numbers where each successive number is the sum of the previous number and some constant d., or arithmetic progressionUsed. Find the value of y in the arithmetic sequence: 25 y 16.

Number sequences are sets of numbers that follow a pattern or a rule. to calculate the geometric mean of the geometric sequence: x y z In the geometric sequence: x y z : y 2 xz y Worked examples 1.

The common ratio is multiplied by the first term once to find the second term, twice to find the third term, three times to find the fourth term, and so on. is a list of numbers or diagrams that are in order. a) Does a geometric or arithmetic sequence best model Mikhails salary in year n Explain how you know.


 0 kommentar(er)
0 kommentar(er)
